Simulating Polar Modulation

Note - this page is work-in-progress so expect changes & additions.

We now have some mathematical and practical insight into polar modulation. We also now have some hints regarding it's bandwidth requirements: under certain circumstances both the amplitude and frequency outputs have a high harmonic content. But a practical circuit implementation will impose some bandwidth constraints. For instance, if we use the si5351 for frequency generation, it's output frequency can only be updated at about 12ksps - imposing an upper bandwith on the frequency output of about 6kHz. We therefore want to understand the consequences of these bandwidth restrictions.

 My maths skills are insufficient for me to carry out a theoretical analysis, and I'm not even sure whether it's possible. For instance I was surprised to find that even analysis of a nontrivial FM modulation quickly becomes intractible  - see here. 

I have therefore sought answers by simulating the algorithm. I've written a C program that internally generates test signals, simulates polar modulation and writes the individual samples into an array. It then displays the fourier transform results of this time-sequence of values. 

The simulation parameters I've chosen are:

• The simulation should test bandwidths of the amplitude and frequency outputs up to 192ksps.
• The carrier frequency should be at least 5x this frequency, or about 1MHz.
• The simulation sampling should be at least 2x this frequency, but make it 4x for safety: ie. 4MHz.

With 256K samples my PC performs a Fourier transform and all other simulation calculations in well under a second. This quick evaluation time nicely allows the spectrum results to be refreshed and viewed whilst interactively changing input parameters. The frequency-width of each of the Fourier transform's output array entry = $\frac{sampling-frequency}{no-of-samples}\approx 15.3Hz$, which is more than enough resolution.

The goal with the simulation code is to remove all possible sources of uncertainty or errors so that the results reflect issues with the core modulation technique only. The Hilbert Transform is nontrival and potentially generates slight amplitude and phase errors in its output. For this reason the Hilbert transform has been removed, and replaced by oscillators that directly generate quadrature phase signals. Also the atan2() and power calculation approximations in Guido's original code are replaced by high accuracy calculations.

The core simulation Pseudocode is:

SAMPLES = 1<<18

Fs = 4e6

dt = 1.0/Fs

float output[SAMPLES]

float outputAmplitude

float freqDelta

float f1 = 1600    // set the modulation signal values

float a1 = 1.0

float f2 = 300

float a2 = 1.0

int AmpSubsampling   = Fs/12000    // for 12kHz amplitude updates

int PhaseSubsampling = Fs/12000    // for 12kHz phase updates

for  t = 0..SAMPLES

// calculate the modulating signal

t1 = 2*PI*f1*dt*t

t2 = 2*PI*f2*dt*t

I = a1*cos(t1) + a2*cos(t2)

Q = a1*sin(t1) + a2*sin(t2)

if ( t mod AmpSubsampling == 0) 

outputAmplitude = sqrt(I*I + Q*Q)

if ( t mod PhaseSubsampling == 0)

float phase = atan2(Q, I);

float dp = phase - prev_phase;

prev_phase = phase;

if(dp < 0)   dp += 2*PI; // phase unwrap

if(dp >= PI) dp -= 2*PI; // reverse phase unwrap

freqDelta = dp * Fs/PhaseSubsampling /(2.0*PI)

carrierPhase += 2.0*PI*(fc+freqDelta)*dt

output[t] = outputAmplitude * cos(carrierPhase)

ApplyWindowingFunction(output)

FFTresult[] = FFT_Transform(output)

power[] = convertResultToPowerIndB(FFTresult)

DisplayAsChart(power)

For the Fourier Transform calculation I use this library - it's open-source, quite fast, the code is easily read, and I was already familiar with it, having used it on other projects. Other libraries might be faster, but raw speed is not critical here.

To simulate various conditions, such as amplitude and phase errors that might be introduced by the Hilbert transform, this core simulation code is supplemented with additional calculations.

When I first wrote this code, I spent some time experimenting with it to give myself confidence that it was both doing what was expected, and that I was able to correctly interprete the results. Some experiments were:

• Temporarily replaced the modulated carrier with pure sinusoid and verified that the result appeared in the predicted FFT "bin".
• Temporarily generated two carriers close together and verified that two peaks were present in the FFT result and at the predicted location.
• Temporarily AM modulated the carrier with a low-frequency sinusoid and confirmed the FFT result was a carrier with two side lobes.
• Reduced and increased the amplitude of the carrier by a factor of 10 and confirmed that the power output changed by 20dB.

Results with 192kHz Sample Rate

Finally, with the sample rate set to 192kHz, I checked the spectrum with two equal amplitude sinusoids, one at 3.5 kHz and the other ranging in frequency from just below 3.5kHz down to just 40 Hz. In all cases the spectrum is virtually perfect:

The horizontal lines are 10dB apart, and the total span is about 12kHz. This is good evidence that the algorithm is working as expected.

Results with 12kHz Sample Rate - slight amplitude delay

The spectrum results are sensitive to the relative delay between the frequency and amplitude outputs. The best results are when the amplitude signal is delayed by approx. 190 master clock ticks (4MHz rate). This corresponds to a delay of a little over half the 12kHz sample clock.

With the sample rate set to 12kHz, and one input set to 3.5kHz, and the other just 130Hz lower, some spurious signals are present at 45dB down:

When the difference frequency increases to 500Hz, many more spurious signals appear:

At a difference frequency of 1000Hz, the spurious signals have continued to grow but the nearby peaks remain about 40dB down:

With a difference frequency of 1500Hz the spurious signal peaks are 30dB down:

When the difference frequency reaches 2000Hz, the spurious signal peaks are 25dB down:

With a difference frequency of 2500Hz, the spurious signal peaks are <25dB down:

Finally, with a difference frequency of 3000Hz, the spurious signal peaks are 20dB down:

A similar pattern emerges with different sample rates - with a higher sample rate and the same difference-frequency the power of the spuri are lower (and vice versa).

Results with 12kHz Frequency Sample Rate, Increased Amplitude Sample Rate

...tbd...

Sensitivity to Amplitude Variation

In the following charts, the frequencies are kept constant at the standard IMD test tones of 700Hz and 1900Hz , and the 700Hz amplitude is varied:

Equal amplitude:

700Hz at 0.9 amplitude:

700Hz at 0.8 amplitude:

700Hz at 0.5 amplitude:

700Hz at 0.1 amplitude:

To Follow (maybe):

• Source of errors arising from lower sampling rate
• Alternative phase unwrapping code?
• Hardware configuration and results of tests on the hardware
• Indications of the algorithm's sensitivity to 
• Hilbert transform phase & amplitude errors
• Delays between the amplitude & frequency outputs
• 
  

 

Guido's SSB Polar Modulation - Analysis and Application

This blog post is "work in progress" and may be updated from time to time. 

The uSDX, is a microprocessor-based Software Defined Transceiver developed by Guido PE1NNZ that has a very interesting technique to generate the SSB transmission. The SSB signal is created by rapidly updating both the output frequency and the output power of a class-E driven Power Amplifier. This design results in a power-efficient and very simple low cost circuit:

That's it - that's the entire transmit circuitry to generate a 5W SSB signal! That's crazy, and so much simpler than typical SSB modulation techniques. I'll call this type of modulation "polar modulation" for reasons that will become apparent later.

Guido's approach is such an attractive idea that the QMX is is also adopting polar modulation.  

This blog post describes my journey learning about polar modulation, how it works, what its limitations are, and how those limitations can be minimised in a practical implementation. The journey I took led me down many blind alleys. I'll try to spare you from these, and to chart a more direct and logical path.

My first step was to explore how it was even possible for polar modulation to modulate typical audio inputs. Understsanding it for a single input tone is easy - the output frequency and output power are just kept constant. For instance, if the transceiver is tuned to 7MHz upper sideband, and a modulating tone of 1kHz is applyed, the radio sets the si5351 CLK2 output frequency to 7.001MHz, and the PA amplitude to a value proportional to the input signal's amplitude. If the frequency or amplitude of the single tone modulating input changes, the output frequency and amplitude are updated accordingly.

When two audio signals, say at 1kHz and 1.9kHz, are applied then the output to the antenna should comprise two frequencies - one at 7.001MHz and one at 7.0019MHz. How is this possible when the transmitter is only capable of generating one frequency at a time? Well, it turns out that it is possible - by varying both the output frequency and output amplitude in exactly the right way. This can be shown mathematically in my blog post here.

I've gone on to confirm in both simulation software and laboratory-style tests that indeed a two frequency output signal can be accurately generated using polar modulation. However there are limitations to this approach which I will summarise here, and will explain later how I arrived at these conclusions.

Summary of Findings

The polar modulation algorithm is nonlinear, and becomes increasingly nonlinear as the amplitudes of two input tones approach each other.  When this happens, as with any nonlinear system, many harmonics are generated and these harmonics are at frequencies that are multiples of the difference-frequency of the input tones. For example, if the input signals are at 1kHz and 1.9kHz, the difference frequency is 900Hz, and there are harmonics at 1800Hz, 2700Hz, 3600Hz, etc.

The harmonics are critical to the correct generation of the RF output. By "magic" the harmonics generated by the frequency variations exactly cancel the harmonics generated by the amplitude variations, resulting in a clean RF signal. Any reduction in the harmonic signals that are caused by bandwidth limitations in either the frequency or amplitude paths will result in spurious signals in the output.

A rough rule of thumb for the case of equal-amplitude input signals is that the bandwidth of the frequency and amplitude paths need to be approximately 5x the maximum expected frequency-difference in order to keep the output's spurious signals more than 30dB below either test tone. For instance, the si5351 can only be updated at about 12ksps maximum (with overclocking the I2C bus beyond spec), and so has a maximum bandwidth of 6kHz. Such a system will show spurious signals of worse than 30dB whenever equal-amplitude dual-tone signals are applied that have a freequency difference greater than $\frac{6kHz}{5}=1200Hz$. However, as will be seen later, improvements are achievable if the amplitude path has a higher bandwidth than the frequency path.

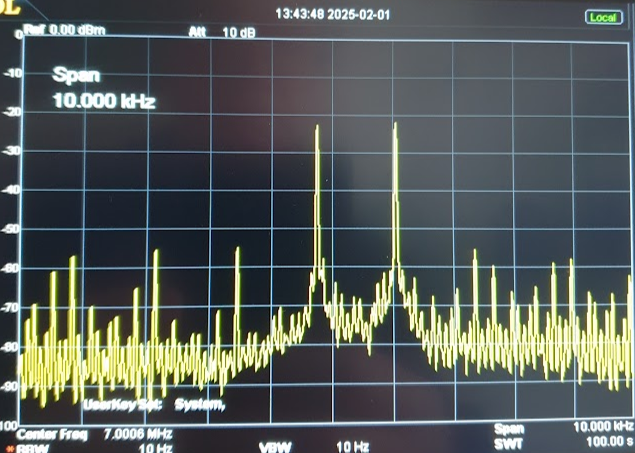
To illustrate the consequences of limiting the bandwith of both paths, here is the simulation result of a 6kHz bandwidth limitation on 300Hz and 1600Hz tones (1300Hz frequency difference) is:

The same test on a laboratory hardware test set-up is:

Note the very strong similarity between the simulated results and real results (allowing for slightly difference horizontal and vertical scales). This is positive evidence of the accuracy of the simulation and my understanding of what is happening.

The Modulation Algorithm

The modulation algorithm at a high level is:

Or in pseudo-code:

modulate(audio): 

(I, Q) = HilbertTransform(audio)

amplitude = sqrt(I*I, Q*Q)

phase = atan2(Q, I)

deltaPhase = phase - lastPhase    // differentiate

lastPhase = phase

if (deltaPhase < 0)  deltaPhase = deltaPhase + 2*PI    // phase unwrapping

if (deltaPhase >PI) deltaPhase = deltaPhase - 2*PI     // phase unwrapping

frequency = deltaPhase * Const + CarrierFrequency

return (frequency, amplitude)

You might rightly wonder what is happening here - this is a huge jump from the description so far and from the mathematical analysis referred to above.

To understand how this algorithm works it helps to first describe:

1. The phasor representation of a sinusoid. 
2. The Hilbert transform, as it applies in this narrow application.
3. Phase unwrapping.

Phasor Representation

The key idea is that, since a sinusoid has both an amplitude and a phase, it can be represented as a vector(arrow) or phasor in a 2D space. The phasor's length is its amplitude, and its frequency is how quickly it rotates around its "tail" in this 2D space. So the tip of a phasor representing a single tone traces a circle in this 2D space. The convention is that the phasor rotates anticlockwise. The horizontal component of this phasor is the level of the signal we see. This is illustrated here.

At any point in time the phasor has a length (the distance from its tail to its tip) and an angle, which is the phasor's angle to the horizontal.

If a signal comprises multiple sinusoids, all the phasors representing these sinusoids are added together tip-to-tail, with each phasor continuing to rotate around its own tail. The result is a new result phasor with its tail at the origin and a tip that traces out a complex pattern in 2D space.

The resulting path could also be traced out by a hypothetical signal generator whose output amplitude and frequency is continually and precisely varied to match. The signal generator's output amplitude is the length of result phasor, and its frequency is the rate of change of the result phasor's angle. This is exactly the calculation being performed in the modulation algorithm!

The Hilbert Transform

The discussion above describes the use of phasors, but the input signal isn't in phasor form. That's where the Hilbert Transform (or Hilbert Filter) comes in - it turns the input into phasor form.

The Hilbert Transform takes an input sinusoid and through "magic" (which I don't understand) outputs two sinusoids I and Q, with Q delayed by exactly 90° compared to I. I and Q are the two dimensions of the 2D phasor space.

The Hilbert Transform is also linear, so if two sinusoids are combined and fed into the Hilbert Transform, the result is exactly the same as feeding each sinusoid into a separate Hilbert Transform and adding the result. ie 𝓗(a + b) = 𝓗(a) + 𝓗(b). This means we can feed our input signal, which comprises multiple frequencies, into the Hilbert Transform and the resulting I and Q signals correctly represent the result phasor described earlier.

Phase Unwrapping

We're almost there! The last issue to deal with is that the phase measurement only gives a raw value between -π and +π. But the actual phase angle of a sinusoid continues to grow without bound. This becomes an issue when we calculate the frequency by measuring the phase's rate of change.

If the raw atan2() phase value is used, the frequency calculation is incorrect each time the phase increases beyond π and jumps back to -π. That's why the following code is needed to "unwrap" the phase and to give the correct phase difference:

if (deltaPhase < 0)  deltaPhase = deltaPhase + 2*PI    // phase unwrapping

It is also possible for the phase be decreasing and for the phase to jump from -π to +π. The code to "unwrap" this case is:

if (deltaPhase >PI) deltaPhase = deltaPhase - 2*PI     // phase unwrapping

"When can the phase be decreasing - that's a negative frequency!" you might ask. Here's an example. Phasor 1 is rotating slowly (say at 100Hz), and Phasor 2 is rotating quickly (say at 1kHz). In the time that Phasor 2 rotates from position 1 to position 2 Phase 1 has hardly moved. The result phasor has moved from pointing to 1 to pointing at 2 - a reduction in phase:

This latter case of phase unwrapping is absent from the uSDX implementation, but appears to be unimportant since it makes little difference to the final signal. Update 29 Mar 2025: this latter case is important after all. Without it, there are increased spuri when the higher frequency tone has slightly lower amplitude than the lower frequency tone.

Studying the appropriate phasor diagrams helps illustrate why the mathematical analysis shows the phase changes so dramatically when the two frequencies are of similar amplitude:

In this diagram, the origin is towards the top, and a trace of the tip of the result phasor is shown over a number of time steps. The low frequency's phasor has its tail at the origin, and its tip is currently in the lower left quadrant. The higher frequency's phasor has it's tail at the LF phasor's tip, and since it is spinning much faster, it is causing the dominant movement; it's tip is the tip of the result phasor. It has similar amplitude to the LF phasor so the result phasor passes very close to, but slightly below, the origin.

For the majority of the result phasor's path, it's angle to the origin only changes relatively slowly from one time step to the next. However, the step from orange to purple, and from purple to red, are very dramatic negative phase changes. This is the dramatic phase change as predicted by the mathematical analysis.

Note that there's nothing special in this description about the LF phasor's location. Another "snapshot" could be taken at a different time where the LF phasor is pointing - say - in the upper right quadrant; exactly the same description of the phase behaviour would apply. In fact different snapshots in time can be thought of as just rotating this image around the origin.

If you now imagine that the HF phasor's amplitude is very slightly larger, then the result phasor would pass very close to, but slightly above, the origin. In this case the dramatic phase changes are positive. A small amplitude change has caused the sign of the phase "blip" to change.

As a final thought experiment, imagine the two amplitudes are exactly the same. In this case the result phasor passes directly through the origin. At one time step it will be on one side of the origin, and on the next time step is will be on the opposite side - a 180° or $\pi$ radians change. The frequency, which is the change in phase over time, will be $\frac{\pi }{dt}$, where dt = the time step duration. If the size of the time step is reduced, then the frequency at the point of crossing increases. This becomes important later - altering the size of the time step alters the result of the frequency calculation - because the calculation depends on a time-derivative.

 Conclusion

This post has hopefully given some intuition behind polar modulation. In a subsequent post I'll investigate the the bandwidth requirements via simulation and a hardware implementation.

Mathematical Analysis of a two-tone SSB Signal

Suppose we have an SSB Transmitter tuned to transmit at carrier frequency $f_c$, upper sideband. With an input audio modulating signal of $f_a$, the transmitter generates an output signal of $f_c+f_a$. For example if the tuned frequency is 7.0MHz and the audio input is 1kHz, a signal at 7.001MHz is generated.

Similarly, two input audio modulating signals $f_a$ with amplitude a, and $f_b$ with amplitude b, the transmitter generates a signal comprising two frequencies, one at $f_c+f_a$  and another at $f_c+f_b$.

The following analysis shows that this 2-frequency output signal can be generated by amplitude-modulating an a single frequency-modulated oscillator. 

The following trigonometric identities are used:

(I): $\cos (x+y)=\cos x\cos y-\sin x\sin y$ -- link

(II) $x\cos \theta +y\sin \theta =c\cos (\theta +\varphi )$ where $c=sgn(x)\sqrt{(x^2+y^2)}$, $\varphi =\arctan (-y/x)$ -- link

(III) ${\sin }^{2}x+{\cos }^{2}x=1$ -- link

We start with the output signal:

$s=a\cos 2\pi (f_c+f_a)t+b\cos 2\pi (f_c+f_b)$

Define ${\omega }_c=2\pi f_c$,  ${\omega }_a=2\pi (f_c+f_a)$ and ${\omega }_b=2\pi (f_c+f_b)$:

$s=a\cos {\omega }_{a}t+b\cos {\omega }_b$

$s=a\cos {\omega }_{a}t+b\cos ({\omega }_{a}t+({\omega }_b-{\omega }_a)t)$

Applying (I): 

$s=a\cos {\omega }_{a}t+b\cos {\omega }_{a}t\cos ({\omega }_b-{\omega }_a)t-b\sin {\omega }_{a}t\sin ({\omega }_b-{\omega }_a)t$

Rearranging:

$s=(a+b\cos ({\omega }_b-{\omega }_a)t)\cos {\omega }_{a}t-b\sin {\omega }_{a}t\sin ({\omega }_b-{\omega }_a)t$

Applying (II), with $x=(a+b\cos ({\omega }_b-{\omega }_a)t)$, $y=b\sin ({\omega }_b-{\omega }_a)t$:

$s=\sqrt{a^2+2ab\cos ({\omega }_b-{\omega }_a)t+b^2{\cos }^2({\omega }_b-{\omega }_a)t+b^2{\sin }^2({\omega }_b-{\omega }_a)t}\cos \left({\omega }_{a}t+{\tan }^{-1}\frac{b\sin ({\omega }_b-{\omega }_a)t}{a+b\cos ({\omega }_b-{\omega }_a)t}\right)$

Applying (III) to simplify the terms under the square root:

$s=\sqrt{a^2+2ab\cos ({\omega }_b-{\omega }_a)t+b^2}\cos \left({\omega }_{a}t+{\tan }^{-1}\frac{b\sin ({\omega }_b-{\omega }_a)t}{a+b\cos ({\omega }_b-{\omega }_a)t}\right)$

This complex equation can be better understood by rewriting it as:

$s=A\cos ({\omega }_{a}t+f(t))$

where:

• $A=\sqrt{a^2+2ab\cos ({\omega }_b-{\omega }_a)t+b^2}$ sets the amplitude of the generated signal, and varies slowly compared to the carrier frequency because ${\omega }_b-{\omega }_a\ll {\omega }_c$, and
• $f(t)={\tan }^{-1}\frac{b\sin ({\omega }_b-{\omega }_a)t}{a+b\cos ({\omega }_b-{\omega }_a)t}$ and varies slowly compared to the ${\omega }_{a}t$ term, again because  ${\omega }_b-{\omega }_a\ll {\omega }_c$.

In other words, combining two input signals results in an output signal with a phase varying around ${\omega }_{a}t$ at the difference frequency ${\omega }_b-{\omega }_a$ and with amplitude A also varying at the difference frequency.

Confidence that the formula is correctly derived can be gained by testing what happens if either of the modulating signals is removed (by setting it's amplitude term - either a or b - to zero. The formula should "collapse" to a single simple waveform. Indeed this is exactly what happens.

The analysis so far draws on "Frequency Analysis, Modulation and Noise", Standford Goldman, 1948 - p160. I believe what follows is novel.

Phase/Frequency Analysis

The full phase term is: $\left({\omega }_{a}t+{\tan }^{-1}\frac{b\sin ({\omega }_b-{\omega }_a)t}{a+bcos({\omega }_b-{\omega }_a)t}\right)$.

The frequency at any instant in time is the derivative of this term with respect to time:

$f=\frac{d}{dt}\left({\omega }_{a}t+{\tan }^{-1}\frac{b\sin ({\omega }_b-{\omega }_a)t}{a+b\cos ({\omega }_b-{\omega }_a)t}\right)$

$f={\omega }_a+\frac{d}{dt}\left({\tan }^{-1}\frac{b\sin ({\omega }_b-{\omega }_a)t}{a+b\cos ({\omega }_b-{\omega }_a)t}\right)$

Deriving the derivative of this second term is beyond my mathematics skills, but thankfully the Derivative Calculator website will do this for us (click the "Go!" button). Here I have defined $m={\omega }_b-{\omega }_a$.

The result is gives is: $\frac{bm(a\cos \left(mt\right)+b)}{b(2a\cos \left(mt\right)+b)+a^2}$

This formula isn't very informative, apart from showing that the output frequency varies with the difference frequency $m={\omega }_b-{\omega }_a$. However, very helpfully, Derivative Calculator also interactively plots the input and derivative values for the input values a, b and m. For example:

Experimenting with different values starts to give some slight intuition about how the amplitude values influence the frequency output. For instance, as the two amplitudes approach each other, the frquency variation grows dramatically:

Finally, when the two amplitudes are equal, there's a discontinuity & the "blip" disappears from the graph view - the negative "blip" is still there and corresponds to the negative edge of the input signal, but it's infinitely narrow and doesn't display:

This tendency for the frequency "blip" to grow without bound as one amplitude approaches the other is extremely important phenomena to understand in later analysis. It will also become apparent that this phenomena changes slightly when we move from the continuous domain to the discrete/sampled domain.

Note that the blue waveform might initially look strange when the amplitude is increased further:

The explanation for the discontinuities in the blue input waveform is that it is a phase measurement and has been displayed to wrap every $2\pi$. The red derivative is displayed correctly and does not show any "blips" at these transitions.

Amplitude Analysis

The amplitude term is $A=\sqrt{a^2+2ab\cos ({\omega }_b-{\omega }_a)t+b^2}$.

Its behaviour can be interactively plotted here.

The most interesting plot is again when $a=b$:

This is a rectified sine wave, as can be demonstrated by setting $a=b=1$. The amplitude term simplifies to:

$A=\sqrt{1+2\cos ({\omega }_b-{\omega }_a)t+1}$

$A=\sqrt{(}2)\sqrt{1+\cos ({\omega }_b-{\omega }_a)t}$

But $sgn(\cos \frac{\theta }{2})\sqrt{\frac{1+\cos \theta }{2}}=\cos \frac{\theta }{2}$  (see link).

So, ignoring the sign term, the amplitude is the shape of a cosine wave.

A rectified sine wave has high harmonic content (see here or here), which will become relevant in later analysis.

Conclusions

This mathematical analysis has derived some equations for both amplitude and frequency shift that appear to correspond to expectations.

The interactive waveform plots give some intuition on the signal behaviour. In particular, when the two tones approach the same amplitude, the frequency shift approaches a discontinuity and values can become very large, and the amplitude waveform approaches a shape that has high harmonic content.

 

Measuring the Si5351 Output Frequency Settling Time

 I'm using the Si5351 programable clock generator in an upcoming project, portions of which are similar to the usdx. For my application, characteristics of the chip that are important, but not documented in the datasheet, are:

• When does the output frequency change in relation to the I2C command that has been issued to update the frequency? Answer: on the second to last low-to-high I2C clock transition of the command that writes the lowest byte of the PLL register.
• How long does the output frequency take to reach the target frequency (its settling time)? Answer: < 1us! Yes, it settles extremely quickly.

This note describes how I measured these characteristics.

For my tests, the Si5351's output frequency is being updated at up to 12k times per second. The I2C bus is overclocked at 800kHz to achieve this update rate. The usdx project implements a frequency-change mechanism where just 5 on-chip 8-bit register values are updated in a single I2S 7 byte transaction. The update is made to alter the PLL frequency. For the purposes of my tests I use the same mechanism, but only need to update 4 registers for the range of frequency changes I'm conducting. Documenting the exact frequency update mechanism is outside the scope of this note, but can be ascertained from the usdx code and reference to AN619.

Note that my tests also showed that the Si5351 output only changes when the lowest byte of 

Test Method

I used a mixer to compare the Si5351's output target frequency against a known reference frequency. The filtered mixer's output is the difference between the input frequencies. So when the two frequencies are the same, or very similar, the output is either constant or changes only slowly. I initially used a filter RC time constant of 1us.

The mixer was built from a 3253 analogue switch. The mixer is very much like a quarter of a Tayloe Mixer.

The fixed frequency could be provided by an external source, but in my case I used a second Si5351 output.  I have relied on the fact that the si5351 can be configured to output 2 frequencies completely independently - each with their own PLL and MultiSynth divider chain (a third output frequency can also be configured, but must share one of the PLLs):

• the target frequency on CLK1 whose frequency will be adjusted by ±4kHz (or more).
• the reference frequency on CLK2 whose frequency is locked and stable throughout a test.

A Pi Pico 2 was used for generating the I2C signals, and output a "high" on a digital pin just before the start of a I2C transaction, and "low" immedately after. An oscilloscope monitored the I2C clock, the digital pin, and the mixer output.

To conduct the measurement, the target frequency would first be set several kHz above or below the reference frequency and allowed to stabilise, and then the target frequency would be set to match the reference frequency.

A typical trace (with the target frequency starting 5kHz above the target frequency, with C = 1nF & RC = 1us) is:

(where yellow = I2C clock, cyan = digital output, blue = mixer output).

The 5kHz difference frequency is clearly visible on the mixer output prior to the frequency change. The flat flat output after the frequency change shows that the target and reference frequencies are now equal.

Zooming in, we see that the mixer output becomes flat (ie target and reference frequencies now equal) within a few microseconds after the second to last low-to-high transition of the I2C clock. The 1us RC time constant of the filter slows the transition:

With C = 100pF, RC = 0.1us there is reduced filtering, but the exact time thta the frequency changed is much clearer:

The frequency transition occurs within 1us.

The tests were rerun using an external Function Generator as the reference frequency. The same results were obtained and verify that there was no unintentional interaction between the two Si5351 outputs.